Rotating Thermal Convection
An interesting variation of Rayleigh-Bénard convection is the case where the sample is rotated about the vertical axis. The rotation speed of the system is defined by the Rossby number (Ro), which is an inverse rotation rate. This system is relevant to numerous astro- and geophysical phenomena, including convection in the Arctic Ocean, in the earth's outer core, in the interior of gaseous giant planets, and the outer layer of the Sun. Thus the problem is of interest in a wide range of sciences, including geology, oceanography, climatology, and astrophysics. Furthermore, the knowledge can be used in industrial applications. An interesting potential is the separation of CO2 from exhaust fumes from power plants. The key question we address is: How does rotation influence heat transport?

Figure 1: Experimental setup at TU Eindhoven
We investigate the influence of rotation by using direct numerical simulations and by conducting laboratory experiments in Eindhoven. In the simulations we solve the incompressible Navier-Stokes and temperature equations with rotation in the Boussinesq approximation in a cylinder. In the experiments a Rayleigh-Bénard convection cell (Figure 1), which is designed to measure the heat transport, is placed on a rotating table to measure the influence of rotation. Figure 2 shows that the heat transfer (the Nusselt number) can increase up to 20% for water when rotation is applied. To get this heat transport enhancement without rotation the temperature difference between the plates should be doubled! This heat transport enhancement is due to Ekman pumping, which leads to an enhanced suction of hot fluid out of the thermal BL and thus in a heat transport enhancement. This process is visualized in figure 3.
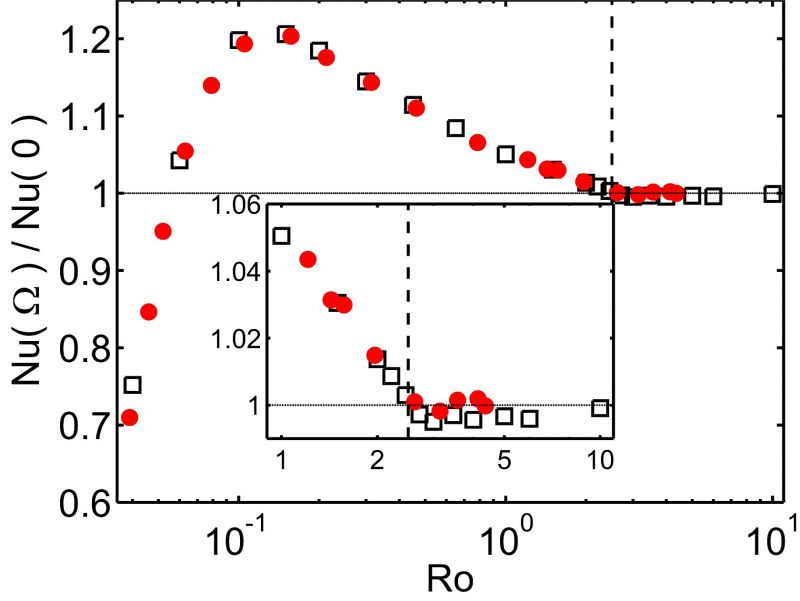
Figure 2: Normalized heat transfer as a function of the Rossby number for Ra = 2.73∙108 and Pr = 6.26. Red solid circles: experimental data from the group of Guenter Ahlers. Open black squares: numerical results.
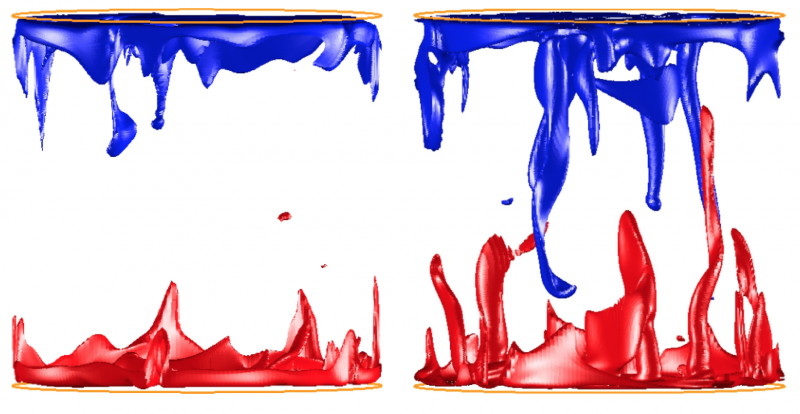
Figure 3: 3D visualization of the temperature isosurfaces showing hot fluid in red and cold fluid in blue for Pr = 6.4 (water) at Ra = 108 and Ro = ∞ (left) Ro = 0.30 (right).
Figure 4 shows that the heat transfer enhancement as function of the Prandtl number has an optimum. This suggests that the effect of Ekman pumping is determined by two different mechanisms. We find that in the low Prandtl number regime the effect of Ekman pumping is limited by the larger thermal diffusivity of the fluid. Therefore the heat that is sucked into the vortices spreads out in the middle of the cell and this limits the effect of the process. In the high Prandtl number regime the kinetic boundary layer becomes thicker with respect to the thermal boundary layer. Because the vortices are formed at the edge of the kinetic boundary layer the temperature of fluid that is sucked into the vortices decreases with increasing Prandtl and this limits the effect of Ekman pumping in the high Prandtl number regime.
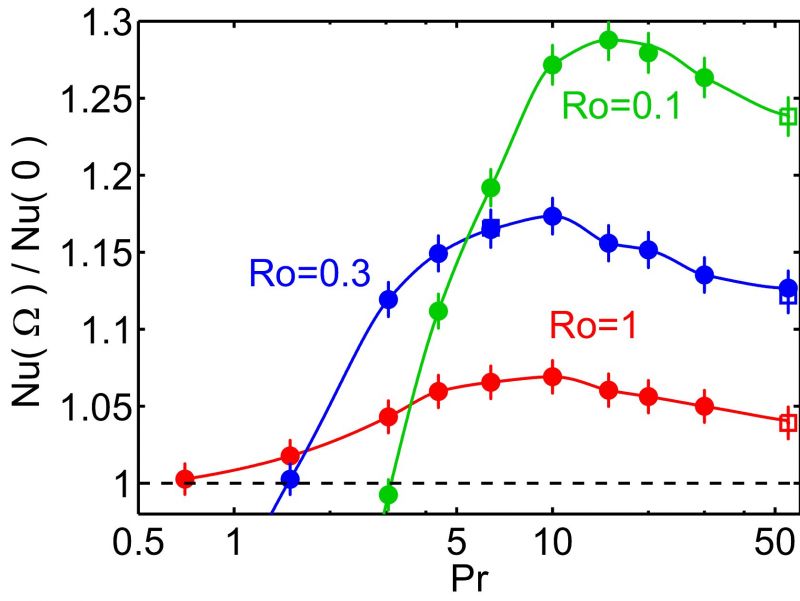
Figure 4: The heat transfer as function of Pr on a logarithmic scale for Ra = 1∙108. Black, red, blue, and green indicate the results for Ro = ∞, Ro = 1.0, Ro = 0.3, and Ro = 0.1, respectively.
Questions we currently address are:
- Influence of rotation on the heat transport
- Influence of rotation on the large-scale circulation (LSC)
- Influence of the aspect ratio
Info: Detlef Lohse
Researchers: Erwin van der Poel, Richard Stevens, Chao Sun, Detlef Lohse.
Collaborators: Herman Clercx (TU Eindhoven), Gert-Jan van Heijst (TU Eindhoven), Guenter Ahlers (UCSB, Santa Barbara)
Embedding: JMBC
Sponsors: FOM
