High Rayleigh number thermal convection
Heat transfer in a Rayleigh- Bénard system can satisfactory be described by the Grossmann-Lohse (GL) theory. In the GL-theory the Prandtl-Blasius boundary layer theory for laminar flow over an infinitely large plate was employed in order to calculate the thicknesses of the kinetic and thermal boundary layers, the kinetic and thermal dissipation rates, and finally these results were connected with the Rayleigh and Prandtl number dependence of the Nusselt number. Because the dynamics of the Rayleigh- Bénard system depends on the location, i.e. in the boundary layers or in the bulk, where most dissipation takes place, the Ra-Pr parameter space can be divided in different regimes as is shown in figure 1.
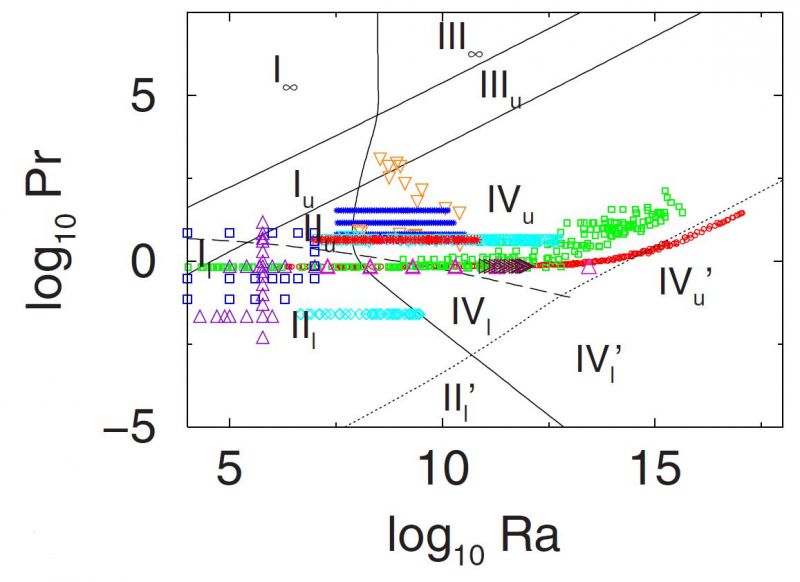
Figure 1: Phase diagram in Ra-Pr plane indicating the different turbulent regimes. The data points indicate where Nu has been measured or numerically calculated.
Experimental and numerical studies of Rayleigh-Bénard convection are complementary to each other. For an accurate experimental measurement of the heat transfer a completely isolated system is needed. Therefore one can not visualize the flow while the heat transfer is measured. In direct numerical simulations one can simultaneously measure the heat transfer while the complete flow field is available. Therefore one can obtain data from simulations that can not be measured experimentally. Because experiments nowadays have reached a high level of accuracy these results can be used to verify the results obtained from simulations. And the simulations can then be used to explain effects observed in experiments. In direct numerical simulations it is very important to resolve all the relevant length scales of the flow and we showed that there is an excellent agreement between experiments and simulations when the resolution is sufficient, see figure 2. In our group we use 2D and 3D direct numerical simulations to analyze the behaviour of the Large Scale Circulation (LSC) in more detail.
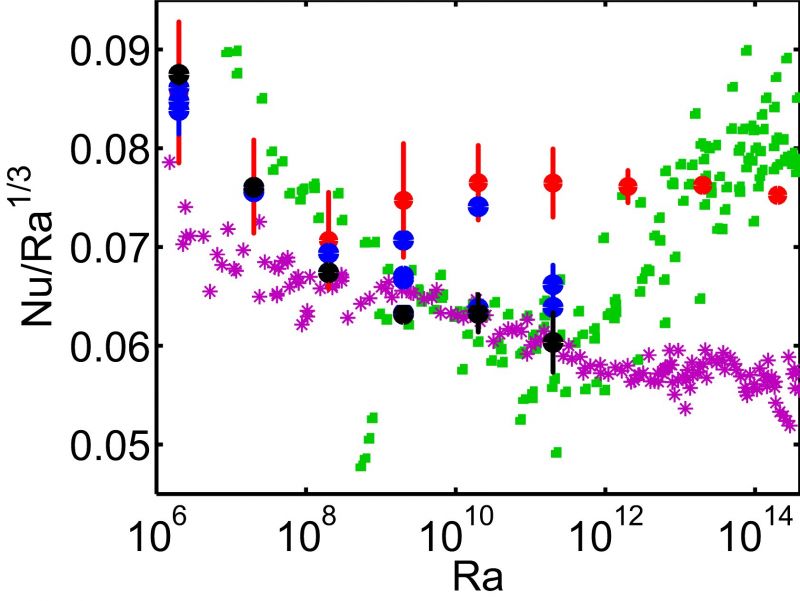
Figure 2: Compensated Nusselt number vs the Rayleigh number for Pr = 0.7. Purple stars are experimental data from Niemela et al. (2000) and the green squares are from Chavanne et al. (2001). The DNS results from Verzicco & Camussi (2003) and Amati et al. (2005) are indicated in red and the present DNS results with the highest resolution are indicated by the black dots. When the vertical errorbar is not visible the error is smaller than the dot size. The results of the underresolved simulations of this study are indicated by the blue dots.
Questions we currently address are:
- The dynamics of the large-scale circulation (LSC)
- Properties of the boundary layers
In other lines of research we work on the influence of rotation and bubbles on the heat transfer in a Rayleigh-Bénard system.
Info: Detlef Lohse
Researchers: Erwin van der Poel, Richard Stevens, Chao Sun, Roberto Verzicco, Detlef Lohse.
Collaborators: Prof. Guenter Ahlers (UCSB, Santa Barbara), Prof. Siegfried Grossmann (University of Marburg), Prof. Federico Toschi (TU Eindhoven), Prof. Andre Thess (Illmenau, Germany), Prof. Penger Tong (Hong Kong, China), Prof. Ke-Qing Xia (Hong Kong, China), Dr. Kazuyasu Sugiyama (Tokyo, Japan), Dr. Enrico Calzavarini (Lyon, France)
Embedding: JMBC
Sponsors: FOM
